
You only need two points to make a line, but for precise in drawings, this time we’ll take three points. This only means that you take x arbitrarily, and your $f(x)$ depends on your choice.Įxample: Draw a graph of linear function: $f(x) = 2x$įirst you have to make your dependant/independant variable table. There are two parts of every linear function, the dependent variable, or $f(x)$ in this case, and independant variable, $x$. For linear functions that graph is a straight line that goes trough the $(0, 0)$ coordinate. A graph is a set of all ordered pairs that satisfy rule of a function. Every function is represented by a graph.
That means that you can take any numbers in the place of $x$ and get yourself an ordered pair of numbers.

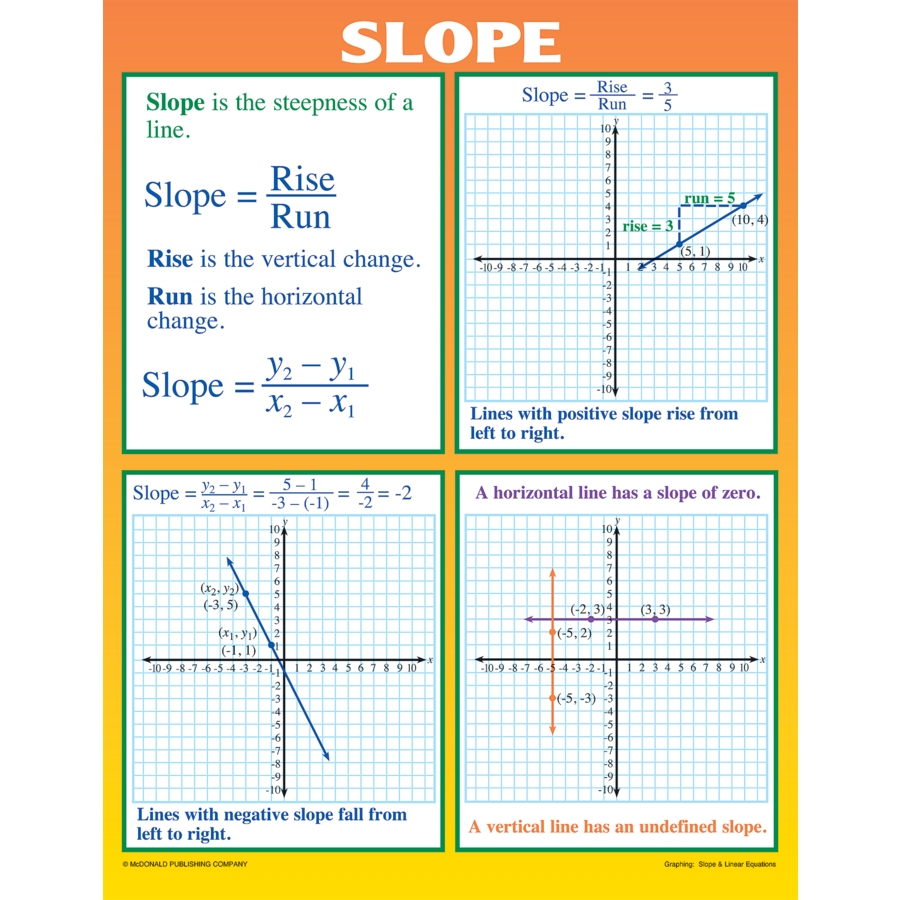
In this rule, $x$ is the changeable variable. In our examples $f (x)$, placed on the bottom of this lessons, will be replaced with $y$. Linear function is a function given by a rule $ f(x) = a \cdot x$, where $a$ is from $a$ set of real numbers. First number indicates where the point is located considering $x$- axis, and second considering $y$- axis. Their intersection will be marked with point $O$, on the $x$-axis, numbers on the left will be negative and on the right positive, and on $y$-axis, numbers below $0$ will be negative and above positive.Ī coordinate is an ordered pair of numbers which tells us where specific point is located. Vertical is called the $y$-axis, and horizontal $x$-axis. We all know what a number line is, but now we’ll have to go a step further and define a number plane.Ī number plane has two axis, horizontal and vertical. We all know that any two points lie on a line, but three points might not.Before we define linear function let’s first define few terms. Still, the move to a geometric property of linear functions is a move in the right direction, because it focuses our minds on the essential concept. In the end it is showing that something is true rather than showing why it is true. It identifies the defining property of a linear function-that it has a constant rate of change-and relates that property to a geometric feature of the graph. It always goes up in steps of the same size, so it’s a straight line. If you go across by 1 on the graph you always go up by $m$, like this: We know that a linear function has a constant rate of change, $m$. When I’ve asked prospective teachers why this is so, I’ve gotten answers that look something like this: The comma indicates that the clause “whose graph is a straight line” is nonessential for identifying the noun phrase “linear function.” It turns the clause into an extra piece of information: “and by the way, did you know that the graph of a linear function is a straight line?” This fact is often presented as obvious after all, if you draw the graph or produce it using a graphing utility, it certainly looks like a straight line.

For example, the function $A = s^2$ giving the area of a square as a function of its side length is not linear because its graph contains the points $(1,1)$, $(2,4)$ and $(3,9)$, which are not on a straight line. Interpret the equation $y = mx + b$ as defining a linear function, whose graph is a straight line give examples of functions that are not linear. In my last post I wrote about the following standard, and mentioned that I could write a whole blog post about the first comma.Ĩ.F.A.3.


 0 kommentar(er)
0 kommentar(er)
